Science
Euclid

Euclid Biography
Euclid or Euclides was a Greek mathematician and geometer, considered one of the great mathematicians of antiquity and father of geometry. He was born in Alexandria in 435 b.C and would die in 265 b.C.. However, there is not much information about his life. The historians say that Euclid would begin his education in Athens, where he acquired his great knowledge of geometry, in the elaborate school of Plato. Later on, Euclid would become professor of his own school in Alexandria, which was the most important in the Hellenic world and in which he would reach the highest recognition as a teacher, in the reign Ptolemy I Sóter, who was the founder of the Ptolemaic dynasty that ruled Egypt and who seek Euclid to teach him and shorten the process to learn mathematics and geometry.
Euclid was the author of different works, such as “Elements”, which is a collection of works of other philosophers such as Hippocrates, and this work would challenge some of the most important literary works in the world, such as the Bible and Don Quixote. This work was made up of 13 books, of which the first 6 made reference to basic flat geometry concepts. From the seventh to the tenth book, Euclid deals with numerical issues such as divisibility, prime numbers, and radicals. In the last three books, he would include topics on the geometry of solids, polyhedra, and circumstantial spheres. Among the most important theorems of Euclid’s work are:
- The sum of the internal angles of a triangle, add up to 180 degrees.
- In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the adjacents, which refers to the Pythagorean theorem.
Besides this, Euclides formulated 5 postulates, which he used as a starting point to explain geometric and mathematical knowledge. These postulates are:
- A straight line segment can be drawn joining any two points.
- Any straight line segment can be extended indefinitely in a straight line.
- Given any straight line segment, a circle can be drawn having the segment as radius and one endpoint as center.
- All right angles are congruent.
- If two lines are drawn which intersect a third in such a way that the sum of the inner angles on one side is less than two right angles, then the two lines inevitably must intersect each other on that side if extended far enough. This postulate is equivalent to what is known as the parallel postulate.
Euclid’s fifth postulate cannot be proven as a theorem, although this was attempted by many people. Euclid himself used only the first four postulates.
The geometrical principles of Euclid were important in areas such as physics, astronomy, chemistry, some engineering and worked as inspiration for the Ptolemaic theory of the Universe in the second century. His ideas also formed an abstraction of reality, because he made assumptions such as that a point has no size so it is assigned a dimension zero or equivalent to zero, a line is a set of points that has neither width nor thickness, only length and assigned a value equal to 1, a surface has no thickness and has dimension 2 equivalent in length and width; to conclude, he said that a solid body, like a cube, has dimension equivalent to 3, length, width, and height.
“Elements” has had more than a thousand editions since the first time it was published in the year 1482, so it is said that Euclid is one of the most read mathematics in history.

Doctor
Sigmund Freud

Biography of Sigmund Freud
Sigmund Freud ( May 6, 1856 and died September 23, 1939) was a neurologist of Czech origin. He was born in Příbor, where his father was a wool trader. Because his father was quite old at the time of his birth and had much older siblings, Sigmund developed great curiosity and asked many questions from a young age. He was very intelligent and skilled from his youth. The economic crisis in the country forced the family to move to Vienna, where they continued to face economic problems due to his father’s lack of employment.
Despite his dislike for Vienna due to these difficulties, Freud lived in this city until one year before his death. For a time, due to his Jewish status, he had to exile himself to London due to the Anschluss, the annexation of Austria to the pan-Germanic project of Greater Germany led by the Austrian chancellor Arthur Seyss-Inquart. Although figures such as Roosevelt and Mussolini intervened, these efforts were unsuccessful and his works were burned.
Despite the persecution, Freud’s family remained faithful to the Jewish community and their customs, although they were not particularly religious since his father leaned towards freethinking, and as a result, Freud had lost his religious beliefs since adolescence. Since graduating with excellent grades from school, an achievement achieved through the efforts of his parents, he showed a lot of interest and motivation for his future. Although he initially inclined towards law, he ultimately decided to study medicine, his intention was not to practice it but to study the human condition with scientific rigor.
After a period at university, he began to focus on biological research, from 1876 to 1882 he worked in the laboratory of physiologist Ernst von Brücke, where he studied some nerve structures of animals and the anatomy of the human brain. During this time he met Viennese doctor Josef Breuer, who was key to his economic and intellectual development. He also met Martha Bernays, daughter of a family of Jewish intellectuals, with whom he later got married.
Beginning of his career
At that time, to assume the responsibilities of being a husband, he began to work as a doctor, putting aside his investigative career without having a true conviction about this decision. However, he managed to acquire the clinical experience necessary to achieve a certain prestige. In that sense, he obtained important jobs in various departments of the Vienna General Hospital, deciding to specialize in neuropathology. In 1884, he conducted a study on the therapeutic use of cocaine, generating various criticisms because he experimented recklessly with people whom he seriously affected. For this reason, his reputation was somewhat tarnished.
In 1885, he began teaching neuropathology at the Medical Faculty of Vienna, and later on, psychoanalysis. His great intellect allowed him to obtain a scholarship to make a study trip for a year in the city of Paris. He spent several months in the neurology service of the Salpêtrière under the direction of Jean-Martin Charcot, then the most important French neurologist. There he learned about the manifestations of hysteria and the effects of hypnosis and suggestion in its treatment.
Beginning in Psychoanalysis
Freud was known for being an extremely jealous man, which caused him several problems and conflicts with his wife. Upon returning to Vienna, Freud opened a private practice as a neuropathologist, using electrotherapy and hypnosis as treatments for nervous diseases. His book written with Josef Breuer on the treatment of hysteria through hypnosis was a great success, entitled Studies on Hysteria (1895).
From that moment on, Freud began to outline his first ideas about psychoanalysis. Freud began speaking about the method of “free association” and at that time many medical friends had preferred to leave him alone. However, this did not prevent him from continuing with his research and emerging psychoanalytic concepts such as the unconscious, repression, and transference. In 1899, he published his famous work The Interpretation of Dreams, edited in 1900. Five years later, he published Three Contributions to the Theory of Sexuality.
Although his work was innovative and admired, it had few followers. However, in 1906 the situation changed; he managed to consolidate a circle of doctors who later became a psychoanalytic society. In 1908, they held the First Psychoanalytic Congress in Salzburg. From that moment on, his recognition was international, he was invited to the United States to give a series of lectures at Clark University in Worcester, Massachusetts, where his thinking had caused great admiration. In 1910, the International Psychoanalytic Society was founded in Nuremberg, led by Freud’s colleague, Jung, who led it until 1914.
Some works and his deterioration of health
In 1916, Freud published Introduction to Psychoanalysis. He continued to write important books such as The Future of an Illusion (1927), Civilization and Its Discontents (1930), and Moses and Monotheism (1939). During this period, he was diagnosed with jaw cancer and had to undergo several surgeries. From then on, his illness often hindered his intellectual production, but he managed to complete several texts. Finally, he lost the battle against his illness on September 23rd, 1939.
Contribution to psychology
The main contribution of Freud to psychology was his concept of the unconscious. Freud believed that a person’s behavior is deeply determined by repressed thoughts, desires, and memories, which can strongly influence their conduct. As a treatment method, psychoanalysis seeks to bring these memories to consciousness to free the individual from their negative influence. Without a doubt, his theories received important criticisms, but they still represent a great contribution to psychology. It is undoubtable that the spread of psychoanalysis revolutionized the view of human beings and its influence extended to fields like philosophy, literature, and the arts.
Notable works
- The Interpretation of Dreams
- Civilization and its Discontents
- Totem and Taboo
- Three Essays on the Theory of Sexuality
- The Ego and the Id
Science
History of Philosophy

History of Philosophy
The history of philosophy is closely linked to the emergence of religions and states in antiquity. Many authors agree that the interest in knowledge reached its peak in Greek cities, with a strong concentration in Athens. Thanks to this convergence, history accounted for figures such as Socrates, Plato, and Aristotle. Over time, the conceptual development of philosophy integrated the disruptive postulates of thinkers like Descartes, Leibniz, and Kant. These philosophers are commonly known as “Classics of philosophy”.
For a long time, philosophy encompassed all aspects of knowledge. However, as knowledge developed, the different sciences acquired their own identity and became autonomous. Nonetheless, philosophy maintained its ability to systematically address the broader and more general concepts about the world and the human being.
Like religion and mythology, philosophy seeks the essence of things, the origin and cause of fundamental phenomena, the explanation of their properties, movements, effects, and purpose. However, philosophy is distinguished by being a rational, coherent, and rigorous search.
“Philosophy is the systematic effort to find answers, to resolve the central mysteries of existence”.
Philosophy is a set of theoretical elements that necessarily reflect the social reality of each historical era and situation. As such a reflection, philosophy is influenced by the contradictory social, economic, and political interests of its time. Even escapism, the attempt to evade society’s reality, can be a way of expressing certain interests.
“Concept, origin, and method of philosophy”
The word philosophy is composed of two Greek words: “phileo” (love) and “sophia” (knowledge or wisdom). Its literal meaning is “love of wisdom“, but it does not convey the main meanings of philosophy. There are different definitions of this discipline, as well as the reluctance of some philosophers to give a precise definition. In addition, the content of philosophy has changed from its inception to the present day. Today, philosophy is understood as a discipline of generalized concepts about the world and the place occupied by human beings in it. It is an attempt to understand the reality and the essence of the things of life and of the human being, to comprehend the meaning and purpose of existence.
Philosophy arises at the beginning of civilization, that is, during the predominance of the Asian mode of production or slavery in China, India, Egypt, and Greece. Specifically, it appears in Greece in the 7th century BC.
In previous social formations, corresponding to the times of the wild state and barbarism, abstract thought was manifested in religious mythology but not in philosophy. The necessary historical and social conditions were a higher degree of productivity of work, the separation of intellectual work from physical work, and the splitting of society into antagonistic classes with different conceptions of the world, life, and relations between human beings.
Tradition holds that mathematician Pythagoras was the first scientist to call himself a philosopher. The dominant concern of thinkers at that time was to explain the phenomena of nature.
The philosophical doctrine of nature was the first form of philosophical thought.
Philosophical method
Plato said that the first virtue of the philosopher is to be amazed. Manuel García Morente observed in 1937 that a person who finds everything natural, easy to understand and obvious can never be a philosopher. It is necessary to be permanently restless, to be interested in everything, to have the attitude of a child, to be rigorous and accurate in thought.
Plato developed the Socratic method and converted it into dialectic (from the Greek “dialegomai”, which means dialogue). This implies not only asking but also dialoguing, and in this process to purify, to subject to criticism and to clarify the ideas that arise from the dialogue.
Aristotle relied on Plato’s dialectic, gave form and structure to the movement of intuitive reasoning and progress in the succession of affirmations and confirmations. The laws of this movement of rational thought, logic, are Aristotle’s philosophical method.
Philosophers have repeatedly focused on the method of philosophy, revaluing the discoveries of their predecessors, enriching them with their contributions, undertaking new paths and relying on the advances that science is conquering. In this way, they continue the incessant and insatiable philosophical search.
Western philosophy dates back to ancient Greece and is divided into four periods:
1. Ancient philosophy
From the 6th century BC to the decline of the Western Roman Empire in the 6th century AD, this period encompasses many theses and arguments always trying to find the ultimate foundation of all things. In this period, there is an attempt to transcend the cosmovisions and mythologies that prevailed at the time. In this first period, a group of philosophers seeks explanations for the origin of the phenomenon of nature, calling this principle Arjé. Some of the outstanding philosophers in this period are:
- Tales of Miletus: (624-543) a.C. – Water
- Pythagoras: (580-504) a.C. – The number
- Parmenides: (540-470) a.C. – The unique being
- Anaximander(611-546) a.C. – The Apeiron
- Anaximenes(588-524) a.C. – The air
- Democritus (460-370) a.C. – The atom
In Ancient Philosophy, two major periods can also be distinguished: Greek philosophy and Roman philosophy. The period of Greek philosophy developed in Ancient Greece from the 6th century BC until the invasion of Macedonia by the Romans in 148 BC. After the invasion, the period of Roman philosophy began, lasting from the 2nd century BC to the 6th century AD. These two periods are further divided into various stages.
Greek philosophy is generally divided into three stages, namely:
- Pre-Socratic philosophy (between the 7th and 5th centuries BC): or philosophy before Socrates.
- Classical philosophy (between the 5th century BC and the 4th century BC): with Socrates, Plato, and Aristotle among its great exponents.
- Hellenistic philosophy (between the 4th century BC and the 2nd century BC): a stage in which the focus of study changed from the world to the individual being.
Roman philosophy is divided into two stages:
- Philosophy of the Republican era (between the 2nd century BC and the 1st century BC): had among its greatest exponents Cicero and Lucretius.
- Philosophy of the high-imperial period (between the 1st century BC and the 6th century AD): in this, philosophy became increasingly independent of politics.
2. Medieval philosophy
The period of medieval philosophy extends from the 5th century AD, with the fall of the Western Roman Empire, to the 15th century, when the Byzantine Empire fell (1453). This period arose from the relationship between Christianity and Greek thought and developed in Europe and the Middle East.
The most discussed topics in this period were the relationship between reason and faith, the nature and existence of God, and the limits between human freedom and knowledge.
The main philosophers of this period were:
- Saint Augustine Of Hippo: (354-430) AD.
- Isidoro Of Seville: (560 – 636) AD.
- Juan EscotoO Erigena: (810 – 877) AD.
- Avicena: (980 – 1037) AD.
- Averroes: (1126 –1198) AD.
- Saint Thomas Of Aquino: (1225 – 1274) AD.
- William Of Ockham: (1280 – 1349) AD.
3. Modern philosophy
The period of modern philosophy covers from the beginning of the Renaissance and the Protestant Reformation to the last years of the 20th century. This period begins with Renaissance philosophy between the 14th and 15th centuries, giving way to modern thought inspired by Descartes and Kant.
In this modern philosophy, at the end of the 17th century and the beginning of the 19th century, German idealism developed, of which we can mention:
- Immanuel Kant: (1724 – 1804) AD.
- Friedrich Hegel: (1770 – 1831) AD.
Other currents of modern philosophy were: rationalism (between the 17th and 18th centuries) and empiricism.
4. Contemporary philosophy
The period of contemporary philosophy develops from the end of the 19th century, the 20th century and the 21st century, also known as the Contemporary Age. It originated in the mid-19th century and continues to the present, seeking to analyze the reality of man in its entirety. This period covers all philosophical thought developed after modern philosophy.
In this contemporary philosophy, two major currents emerge:
- Continental philosophy (from Europe)
- Analytic philosophy (from the Anglo-Saxon world)
Each philosophical current gives rise to different schools and traditions. For example, from continental philosophy there are derived existentialism, post-structuralism, social constructivism, critical theory and postmodern philosophy. In analytical philosophy, on the other hand, we find schools and currents such as logical positivism (logical empiricism or neopositivism), naturalism, experimental philosophy, philosophy of language and pragmatism.
Among the main philosophers of contemporary philosophy of the 19th century, some of them can be highlighted:
- August Comte: (1798 – 1857) AD.
- Soren Kierkegaard: (1813 – 1855) AD.
- Karl Marx(1818 – 1883) AD.
- Friedrich Nietzsche: (1844 – 1900) AD.
- Edmund Husserl: (1859 -1938) AD.
- Gottlob Frege: (1848-1925) AD.
In the 20th century, philosophy would continue with philosophers such as Sigmund Freud, Ernst Mach, Jean-Paul Sartre, Bertrand Russell, Simone De Beauvoir, Max Horkheimer, Theodor Adorno, Albert Camus, Karl Jaspers, Michel Foucault, Gilles Deleuze, Jacques Derrida, Ludwig Wittgenstein and Karl Popper.
In conclusion, in the philosophical tradition from its origins to the present day, there have been a large number of philosophers and philosophical movements, so it was argued some of the main characteristics of the philosophical history.
Science
The history of the atom
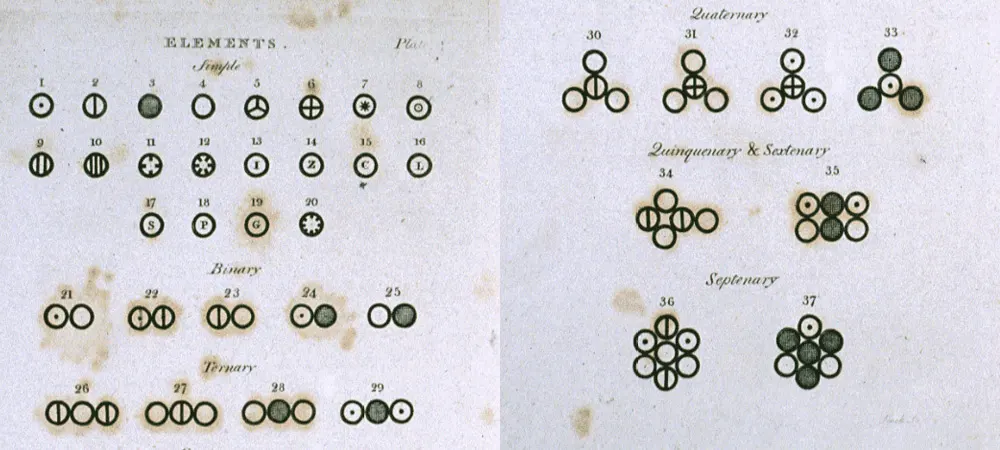
The history of the atom
The history of the atom begins 450 years before Christ with the assertions postulated by the Greek philosopher Democritus of Abdera. The philosopher was interested in the discovery of essential substances that contain all substances. He claimed that matter could be indefinitely divided into increasingly smaller particles until reaching the most indivisible point of that matter, which Democritus called atoms, a word that in Greek means inseparable. So, matter was composed of atoms and these were inseparable, so Democritus made a distinction between previous thinkers, who named atomic elements to elements such as water, air, and fire. Democritus claimed that these were not atoms in themselves, but were composed of thousands of them.
In short, Democritus supposed that all matter is composed of solid, indivisible and invisible to the human eye particles, the famous atoms. Although this philosopher interested in physical and chemical processes never had a true proof that proved the existence of the atom. We can affirm that he was the first person to talk about this and consolidate an atomist conception, known today as the Discontinuity of Matter, generating a long debate with the passing of the centuries.
The philosopher Leucippus of Miletus based his idea of the rational origin of the universe on the atom, claiming that the universe was made up of thousands of indivisible particles that came together after an event similar to a whirlwind. Epicurus of Samos, a philosopher from Athens, with his doctrine of nature, claimed, reworking Democritus’s version, that the formation of the universe could have responded to a process of chance, in other words, the probability that atoms would suffer deviations in their trajectory, colliding with each other.
It took several centuries for John Dalton, known as the father of atomic theory, to be born in 1776. He was born in the United Kingdom, specifically in Cumbria. From the age of 12, he showed his intelligence. As a young man, he was interested in meteorology and from there his attraction to certain chemical phenomena exploded. Dalton’s postulates marked a major change in knowledge about atoms and their behavior.
In that sense, the scientist claimed that matter is composed of indivisible atoms, this statement was not very new. But, in addition, he added that atoms have an immutable character, that is, they can never be transformed into each other, what has variable value are chemical combinations because they are made up of identical molecules and these in turn by atoms. Thanks to an endless number of experiments carried out by Dalton, the Dalton Atomic Theory was established.
The mentioned theory helped to calculate the atomic weight of the elements, such as the gaseous elements. He discovered the atomic masses of several elements by relating them to the mass of hydrogen. These discoveries were presented on October 21, 1803 during a conference at the Manchester Literary and Philosophical Society. Later, the disquisitions were reflected in his famous book A New System of Chemical Philosophy, published in 1808.
In this text, the following general statements can be highlighted: matter is composed of atomic particles, indivisible and indestructible, atoms of the same element are equal, as well as their weight and qualities, atoms do not divide even when they combine through chemical reactions, atoms of different elements can combine and form compound atoms, finally, chemical compounds are born from the union of atoms of two or more different elements. Many of Dalton’s statements were challenged or reaffirmed.
In the future, Michael Faraday reformulated several of Dalton’s proposals. In 1883, he discovered that the flow of electric current from one substance to another produces certain chemical changes, indicating the existence of a relationship between electricity and matter, ensuring that atoms must have an electric structure that supplies the appropriate amount of electric current to the weight of the decomposed chemical substance.
En el año de 1906 sale a la luz el Modelo Atómico de Thomson, que claramente invalidaba el anterior Modelo Atómico de Dalton ya que este no reflexionaba sobre la estructura interna del átomo. El físico británico Joseph John Thomson se valió del uso de los rayos catódicos dispuestos en un tubo de vacío que eran desviados al aplicar un campo magnético para obtener las pruebas para dar a luz este modelo.
The Thomson atomic model postulates that: The atom has negatively charged electrons embedded in a sphere of positive charge, these electrons are uniformly distributed throughout the atom, the atom is neutral so that the negative charges of the electrons are offset by the positive charge, the electrons can be extracted from the atom of any substance. Thus, Thomson represented the atom with a static model, in which the electrons were fixed within the positive mass, this model was approved by the scientific community because it allowed to explain qualitatively phenomena such as the emission of light by atoms, although later facts modified this hypothesis.
Ernest Rutherford was the one who modified Thomson’s model, who in 1911 considered that in the central nucleus of the atom there is the positive charge and mass; while around there are electrons spinning at high speed. On the other hand, he discovered that the nucleus has a crust and a nucleus, the electrons that spin do so in the crust of the atom around the nucleus; this region is small and is located in the center of the atom that has the positive charge.
Just two years later, Niels Bohr, studying Rutherford’s model disciplinely, deepened the way in which electrons were kept under a stable orbit around the nucleus without radiating energy, also thanks to the quantum number n, he was able to assure that first: there is a distance between the orbit and the nucleus; second that not all electrons circulate through all orbits and third he calculated the radius of the orbit. Bohr also explained why atoms showed characteristic emission spectra and how electrons can emit or absorb energy during jumps from one orbit to another. Shortly thereafter, the Sommerfeld model came out, based on Bohr’s, formulating contributions to relativistic mechanics indicating that electrons travel at speeds close to that of light. It can also be highlighted that for Sommerfeld, the electron is basically an electric current. In 1924, the Schrödinger model, formulated by Erwin Schrödinger, came to light, which as an innovation takes into account the four quantum numbers: n, i, m, s. to affirm that in an atom there are no electrons with the four quantum numbers equal.
In the 60s American physicists Murray Gell-Mann and Georg Zweig detected a subatomic particle called a quark. In the 21st century a team of scientists carried out experiments in the Large Hadron Collider found the pentaquarks. This discovery of the subatomic particle helps to better understand the constitution of ordinary matter, neutrons and protons.
Science
The history of biology

The history of biology
The origin of biology dates back to Greek philosophy, when the natural sciences were introduced. Hippocrates was the first to give a biological concept of life, and Aristotle is recognized as the first to classify animals. Aristotle was one of the greatest naturalists of ancient times and his greatest interest was living nature. He was the first great biologist of Europe and formulated the principle that all organisms are adapted to the environment in which they live. In addition, he stated that nature does not release energy unnecessarily, that is, it is parsimonious.
Aristotle, a biologist two thousand years ahead of his time: Some biological observations made by Aristotle took centuries to be confirmed by naturalists, especially those referring to the habits of aquatic animals. Aristotle pointed out that the male of the catfish guarded the eggs laid by the female until the fry were born. For a long time, Aristotle’s assertions, fruit of his observations, were considered to be fables.
The origin and evolution of Biology
Biology can be defined in a seemingly simple but precise way as the science that studies life. The historical discussion revolves around what life is.
 The etymology of the word Biology is formed by the combination of the Greek terms: “bios” which means life and “logia” which means science.
The etymology of the word Biology is formed by the combination of the Greek terms: “bios” which means life and “logia” which means science.
The first samples of biology go back to the study of living beings and their vital manifestations from ancient times to our time. In this sense, the first classifications of living beings were made according to practical criteria considering usefulness and risk. It was not until the work of Linné (Carlos Linneo) saw the light, in the seventeenth century (Species Plantarum), when a “nature” classification was found.
Although the concept of Biology as a science was born in the 19th century, it studies all aspects or characteristics of living beings such as their chemical composition, reproduction, growth, metabolism, cellular organization and movement.
During the 18th and 19th centuries, biological sciences, such as botany and zoology, became scientific disciplines. It was during these centuries that Lavoisier and other physical scientists began to unite the animate and inanimate worlds through physics and chemistry. Explorer-naturalists, such as Alexander von Humboldt, expanded the fields of science by investigating the interaction between organisms and their environment, initiating biogeography, ethology, and ecology. Later, the cell theory provided a new appearance on the foundations of life.
The history of biology is divided into three major stages:
Ancient: theories and discoveries made from prehistory to the Middle Ages. In this sedentary stage of life, man began to observe phenomena of nature such as changes in seasons, tides, rainfall, all this attributed to the action of different gods; this way of explaining natural phenomena through religion and mythology lasted until the 6th century BC. Period in which several Greek philosophers called naturalists appeared, among them were Tales of Mileto, Anaximander, Pythagoras, Jenofanes of Colophon and Parmenides of Elea. Then the first documents of biology appeared, many of them attributed to Hippocrates and he is remembered for the Oath. Aristotle was considered the Father of Zoology; and Galen, last doctor of antiquity, as father of Anatomy.
Modern: With the Renaissance, this era of Biology began that lasted until just before the second decade of the 20th century. Here great biological changes were defined and some apparatuses and tools were invented that made research more optimal. Among the most important advances made in this stage is the invention of the microscope, with which biological structures that were not possible to see at first sight began to be observed.
Modern biology is based on several unifying themes, namely:
- The Cell Theory.
- The Theory of Evolution by Natural Selection of Darwin and Wallace.
- Mendel’s Laws.
- The Chromosomal Theory of Inheritance.
- Crick’s Central Dogma on the flow of information.
This stage was also characterized by the use of an experimental work method and the attempt to relate cellular structures to their function. New fields of Biology emerged such as Microbiology and Genetics.
Within this period, some famous researchers stand out in the establishment of the importance of the cell as the fundamental anatomical unit of all living organisms. Among them are:
- Robert Hooke: the first scientist to use the word “cell”.
- Robert Brown: Established in 1831 that all cell types have a nucleus.
- Matthias Schleiden and Theodor Schwann: In 1838, both biologists established that the cell was the fundamental anatomical and structural unit of all living beings. These would be two of the postulates of the Cell Theory.
- Rudolf Virchow: Proposed the third postulate of the Cell Theory by ensuring in 1858 that the cell is the unit of origin.
Other important researchers of this time were Charles Darwin (theory of evolution); Louis Pasteur (founder of microbiology and creator of the rabies vaccine); Gregor Johann Mendel (Mendel’s laws) and Carlos Linneo (classification of organisms, system of nomenclature).
Molecular: This is the current moment, based on the basis of cellular constitution. Molecular life, which can be called biology of our time in a way, begins in 1920. The invention of the electron microscope, technological advances have made possible great achievements in the different fields of biology, highlighting in particular what has been achieved at the level of Genetic research.
At the beginning of the 20th century, the rediscovery of Mendel’s work led to the rapid development of genetics by Thomas Hunt Morgan and his students, the combination of population genetics and natural classification in modern evolutionary synthesis during 1930. New sciences developed rapidly, especially after James Watson (American biologist) and Francis Crick (British biologist) discovered the structure of DNA in 1953. At the end of the 20th century, new fields such as Genomics and Proteomics inverted this trend, with organic biologists using molecular techniques and investigating the interaction between genes and the environment.
Current
In the 21st century, biological sciences contributed as new and classic disciplines previously differentiated as physics in research fields such as biophysics. Advances were made in analytical chemistry and physical instrumentation, optical components, networks, satellites, and computing power for data collection, storage, visualization, and simulation. All of these technological advances allowed for the theoretical and experimental search for molecular biochemistry, biological systems, and ecosystem science. This made global entry possible for the improvement of measurements, complex simulations, analysis, observational content of data over the internet. New research fields in biological sciences emerged such as “bioinformatics” (application of computational technologies to the processing and observation of biological data). “Theoretical biology” (conceptual characterization of biological problems). “Computational genomics” (the use of computational analysis to interpret the biology of genome sequences). “Astrobiology” (combines biology and astronomy to study the origin, evolution, distribution, and future of life in the universe) and “Synthetic biology” (the synthesis of biomolecules, the belief that studies the chemical composition of living beings).
The current moment is that of biotechnology, genetic engineering, and genomics. Contributions from scientists, biochemists, physicists, and engineers are important within this stage, such as Max Perutz and John Kendrew, fundamental in the rapid development of structural biology; E. O. Wilson (biologist, father of biodiversity); Niels Kaj Jerne (immunologist, co-author with Frank Macfarlane Burnet of the clonal selection theory); Moto Kimura (author in 1968 of the neutralist theory of molecular evolution); Paul Berg (biochemist, obtained the first artificial DNA molecule, recombinant DNA, in 1972); Frederick Sanger (discoverer of the structure of insulin); Walter Gilbert (Nobel Prize in Chemistry for his studies on the structure and evolution of DNA sequences) and Carl Woese (creator of the new molecular taxonomy based on the comparison between species of the 16s and 18s mitochondrial RNA.
Some branches of Biology are:
- Anatomy
- Anthropology
- Bacteriology
- Biophysics
- Marine biology
- Biomedicine
- Biochemistry
- Biotechnology
- Botany
- Cytogenetics
- Cytochemistry
- Ecology
- Entomology
- Ethology
- Evolution
- Physiology
- Genetics
- Molecular genetics
- Histology
- Immunology
- Microbiology
- Parasitology
- Paleontology
- Taxonomy
- Virology
- Zoology
Doctor
Willem Einthoven

Willem Einthoven Biography
Willem Einthoven (May 21, 1860 – September 28, 1927) Physiologist and physician. Nobel Prize in Medicine in 1924. He was born in Semarang, Indonesia. He is well known for his contributions to the development of the electrocardiograph and its clinical application. His father died when they lived in Java, so Willem moved to the University of Utrecht to study medicine.
After finishing his studies he obtained the position of professor at the University of Leiden to deal with the positions of physiology and histology. He took the opportunity to advance an important work in the field of research. He quickly showed himself as a reputable scientist, participated in numerous international scientific forums and the best thing is that by managing several languages he could communicate his ideas faithfully without the need for translators.
For several years he experimented with the rope galvanometer and its utility for the registration of cardiac potentials, and the results obtained were published in an article in the year 1901. Five years later, he masterfully described the clinical applications of the electrocardiogram in Telecardiogramme (1906). After that, he published another article that laid the foundations for the development of this important tool in cardiology analysis. His investigative work was carried out simultaneously with his work as a professor.
Thanks to his work, the galvanometer was used to measure the differences in electrical potential during systolic and diastolic heart contractions and reproduce them graphically. This procedure is known as an electrocardiogram.
Later, he was interested in analyzing how healthy hearts worked and then defining a reference frame, through which attention was paid to the deviations caused by the disease. To sum up, he revolutionized the study, diagnosis, and treatment of cardiac pathologies. In his honor, the lunar crater Einthoven bears his name.



















































